Understanding Significant Digits and Measurement – A Comprehensive Guide

Have you ever wondered why a scientist might report a measurement as 10.00 grams instead of just 10 grams? The answer lies in the concept of significant digits, a fundamental aspect of scientific measurement that ensures accuracy and clarity in communication. While it may seem like a detail, understanding significant digits is crucial for anyone working with measurements, whether it’s in a laboratory, a manufacturing facility, or even in everyday life.

Image: studylib.net

This guide will demystify the world of significant digits and measurement, providing a comprehensive explanation of the rules, principles, and practical applications. We’ll explore the historical context, delve into the key concepts, and illustrate these principles with real-world examples to solidify your understanding.

The Foundation: What are Significant Digits?

Simply put, significant digits, also known as significant figures, represent the meaningful digits in a measurement. These digits convey the precision of a measurement, indicating how accurately a quantity has been determined. They provide a clear picture of the reliability of the data, enabling scientists and engineers to make informed decisions.

For instance, a measurement of 10.00 grams suggests higher accuracy than a measurement of 10 grams. The additional zeroes in 10.00 indicate that the measurement was taken to the hundredths place, making it more precise and reliable. This distinction might not seem significant at first, but in scientific and engineering contexts, even small differences in precision can have substantial consequences.

Understanding the Rules: Deciphering Significant Digits

To accurately determine the number of significant digits in a measurement, we follow a set of well-defined rules:

1. Non-Zero Digits

All non-zero digits are considered significant. For example, the number 345 has three significant digits.

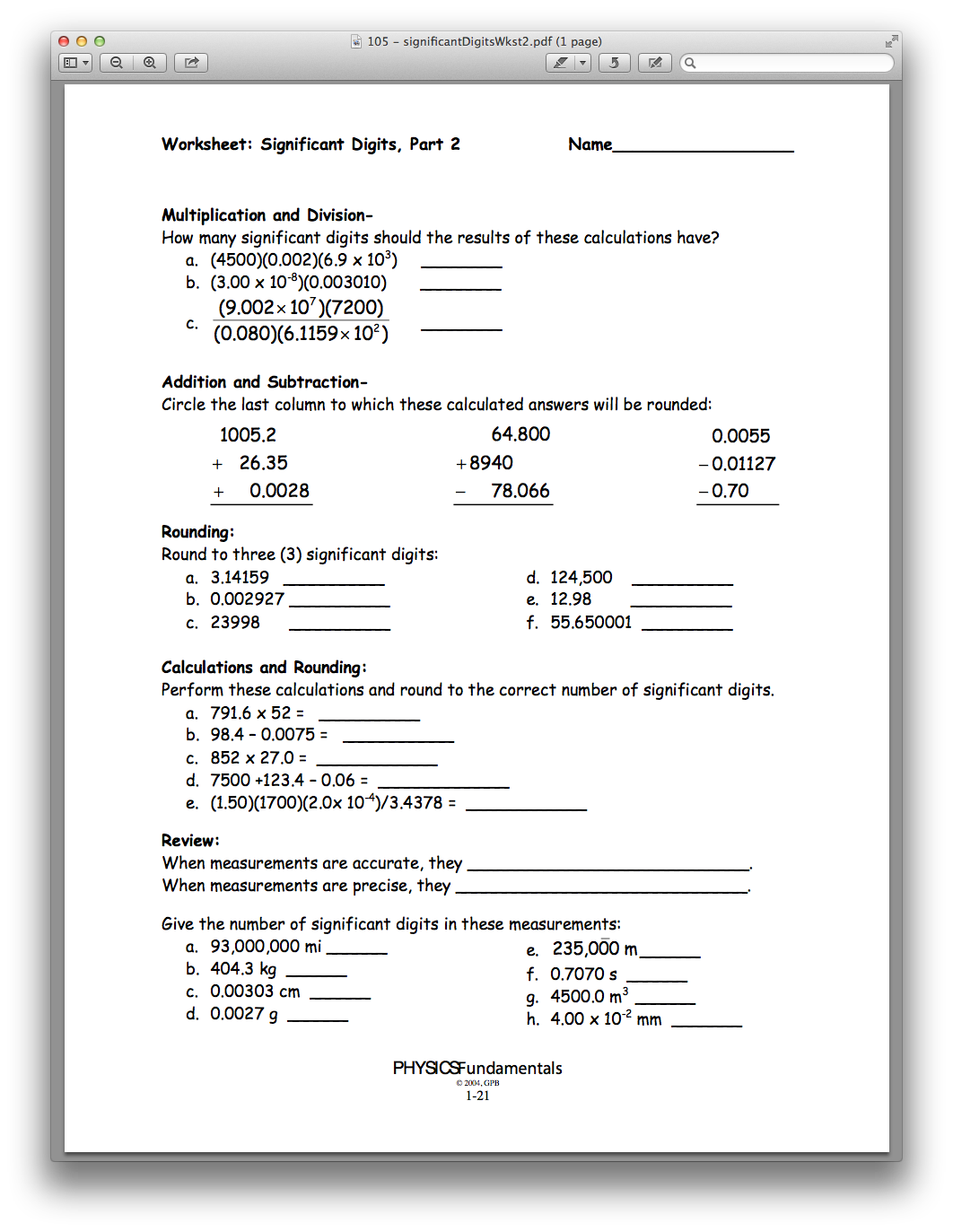
Image: ivuyteq.blogspot.com

2. Zeros Between Non-Zero Digits

Zeros located between non-zero digits are always significant. For instance, 205 has three significant digits, and 1.008 has four significant digits.

3. Leading Zeros

Leading zeros, which appear before the first non-zero digit in a decimal number, are not significant. These zeros merely serve as placeholders. For example, 0.005 has only one significant digit (5).

4. Trailing Zeros

Trailing zeros after a decimal point are always significant. For example, 2.00 has three significant digits, and 10.000 has five significant digits. Trailing zeros in whole numbers can be ambiguous unless specifically indicated otherwise.

5. Exact Numbers

Exact numbers, defined as quantities with infinite precision, do not influence the number of significant digits in a calculation. Examples of exact numbers include counting numbers (like the number of students in a class) and defined values (like the number of inches in a foot).

Significant Digits in Calculations: Maintaining Precision

When performing calculations with measurements, it’s crucial to maintain the appropriate number of significant digits to ensure the result reflects the precision of the original data. Here’s a breakdown of the rules for calculations:

1. Addition and Subtraction

The result should have the same number of decimal places as the measurement with the fewest decimal places. For example, 2.55 + 1.2 = 3.75, which rounds to 3.8 because 1.2 has only one decimal place.

2. Multiplication and Division

The result should have the same number of significant digits as the measurement with the fewest significant digits. For example, 12.50 x 3.2 = 40.00, which rounds to 40 because 3.2 has two significant digits.

3. Rounding

When rounding a number, consider the digit immediately to the right of the last significant digit. If it’s 5 or greater, round the last significant digit up. If it’s less than 5, leave the last significant digit as is.

Real-World Applications: Why Significant Digits Matter

Understanding significant digits is not just an academic exercise; it’s essential for accurate and reliable measurements in various fields. Here are some examples:

1. Engineering

Engineers rely on precise measurements to design and build structures, machines, and other complex systems. For example, in bridge construction, even a slight miscalculation caused by improper handling of significant digits could compromise the structural integrity and lead to catastrophic consequences.

2. Chemistry

Chemists carefully measure the quantities of reactants and products in chemical reactions. Understanding significant digits ensures that the results of these experiments are reliable and reproducible. This is crucial for developing new materials, pharmaceuticals, and other chemical products.

3. Healthcare

In healthcare, accurate measurements are critical for diagnosing and treating patients. For example, the dosage of medication is often determined based on weight and other measurements, making accurate significant digit calculations vital for patient safety.

The Future of Measurement: Ongoing Advancements

The field of measurement continues to evolve, driven by advancements in technology and increased demand for precision. New tools and techniques are constantly being developed, leading to more accurate and reliable measurements. For example, the development of atomic clocks has revolutionized timekeeping, while advanced imaging techniques have improved the precision of measurements in fields like medicine.

Significant Digits And Measurement Answer Key

Conclusion: Embracing the Significance of Digits

In conclusion, understanding significant digits is not only essential for scientists and engineers but also beneficial for anyone working with measurements, as it ensures accuracy, clarity, and consistency in communication. By adhering to the established rules and principles, we can confidently interpret and utilize measurements in various fields, contributing to accurate and reliable data analysis. As technology continues to advance, the importance of precise measurement and understanding significant digits will only become more pronounced.