Lesson 11.5 Practice A Geometry Answers – Mastering Geometric Concepts

Remember that thrilling moment in geometry class when your teacher introduced the concept of congruent triangles? You were tasked with proving two triangles were identical, their sides and angles perfectly matching. The challenge sparked a sense of curiosity, a desire to understand the intricate world of shapes and their relationships. That’s the beauty of geometry; it’s a journey of discovery and problem-solving. And Lesson 11.5 Practice A in your geometry textbook, probably a collection of practice problems focusing on congruent triangles, acts as a stepping stone on that journey.

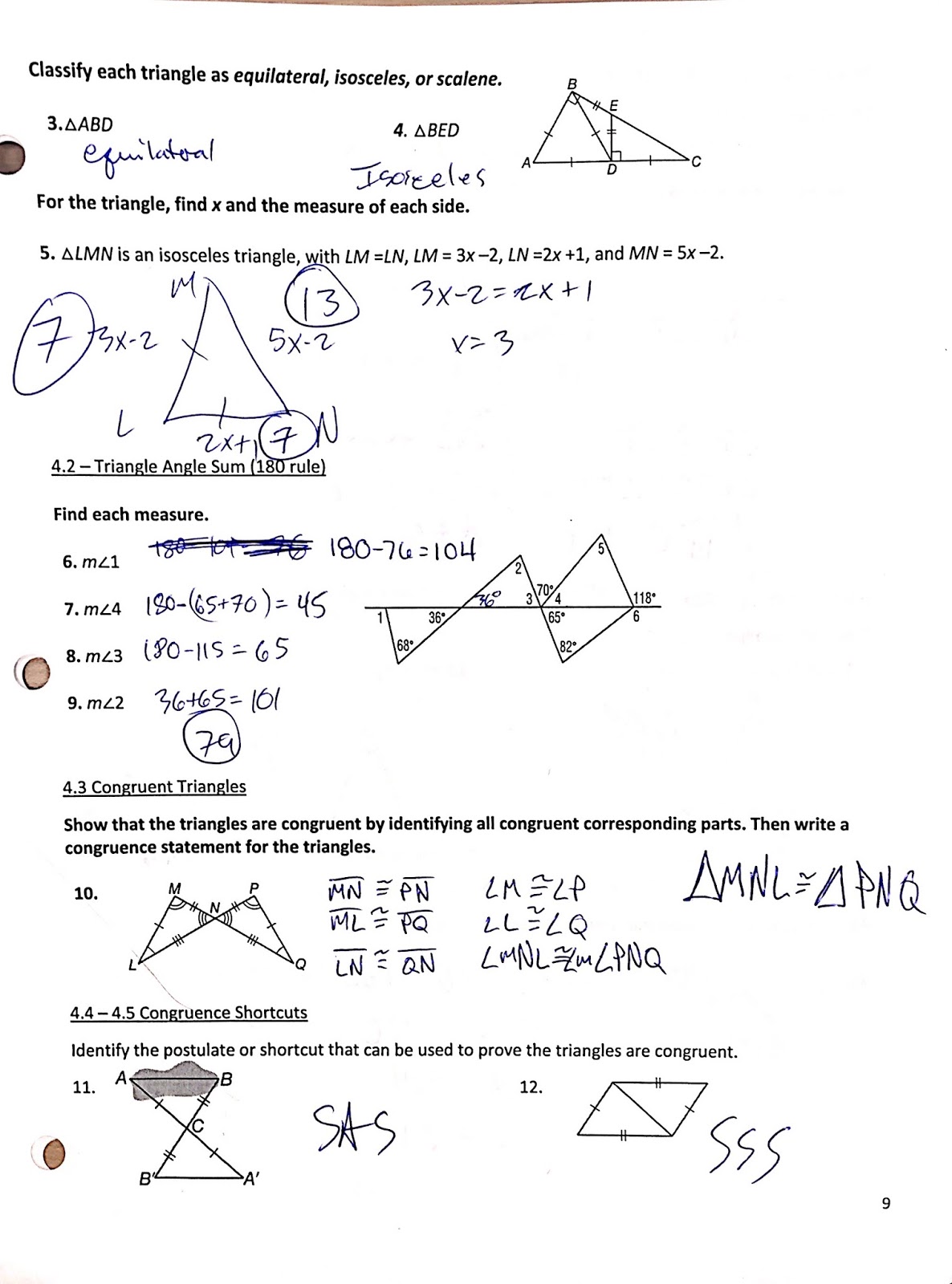
Image: printablelibwaneer.z19.web.core.windows.net

But let’s face it, sometimes navigating those practice problems can feel like navigating a maze. You might stare at the diagrams, wondering where to start, how to apply the postulates and theorems you’ve learned. That’s where this post comes in! We’ll delve into the world of Lesson 11.5 Practice A geometry answers, providing clear explanations, step-by-step solutions, and essential tips to help you conquer those problems and deepen your understanding of congruent triangles.

Understanding the Concept of Congruent Triangles

In geometry, congruent triangles are like identical twins. They have the same shape and size, meaning their corresponding sides and angles are equal. Imagine two pieces of paper cut into the same triangle shape. You can perfectly overlap them, and they fit together seamlessly. That’s congruence in a nutshell! But how do we determine if two triangles are congruent? That’s where the postulates and theorems come in. These are like rules or formulas that tell us the conditions for proving congruence.

Some of the most common postulates and theorems you’ll encounter in Lesson 11.5 Practice A include:

• SSS (Side-Side-Side): If three sides of one triangle are congruent to three sides of another triangle, then the triangles are congruent.
• SAS (Side-Angle-Side): If two sides and the included angle of one triangle are congruent to two sides and the included angle of another triangle, then the triangles are congruent.
• ASA (Angle-Side-Angle): If two angles and the included side of one triangle are congruent to two angles and the included side of another triangle, then the triangles are congruent.
• AAS (Angle-Angle-Side): If two angles and a non-included side of one triangle are congruent to two angles and a non-included side of another triangle, then the triangles are congruent.

Exploring Lesson 11.5 Practice A Geometry Answers

Let’s dive into the details of Lesson 11.5 Practice A and see how these postulates and theorems are applied. Each practice problem in this section typically presents a pair of triangles, and your goal is to determine if they are congruent. If they are, you need to identify the postulate or theorem that justifies your answer. Here’s how to approach those problems:

1. Identify Corresponding Parts: Look at the triangles and accurately identify the corresponding sides and angles. Mark them with congruent symbols (like little tick marks for sides and arcs for angles).

2. Apply the Postulates/Theorems: Analyze the given information – what sides and angles are congruent? Do they match the requirements of any of the postulates or theorems? If yes, then you can conclude the triangles are congruent and specify the reason.

3. Show Proof: Often, you will need to write a formal proof. This is basically a step-by-step explanation of how you determined the triangles are congruent. Start with the given information, then cite postulates or theorems, and finally state your conclusion.

Tips for Success

Here are some handy tips to conquer those practice problems and make Lesson 11.5 Practice A your friend:

• Draw Diagrams: Always draw a clear diagram of the triangles—even if one is provided. This visual representation will help you understand the relationships and identify corresponding parts.
• Label Thoroughly: Label the sides and angles with letters or symbols to make it easier to track them during your analysis and proof-writing.
• Practice, Practice, Practice: The more practice you get, the better you’ll become at recognizing the conditions for congruent triangles and applying the proper postulates/theorems. Don’t shy away from challenging problems; they’re your best learning tools.
• Seek Help: If you get stuck, don’t hesitate to ask for help from your teacher, tutor, or classmates. They can offer valuable insights and help you overcome any obstacles.

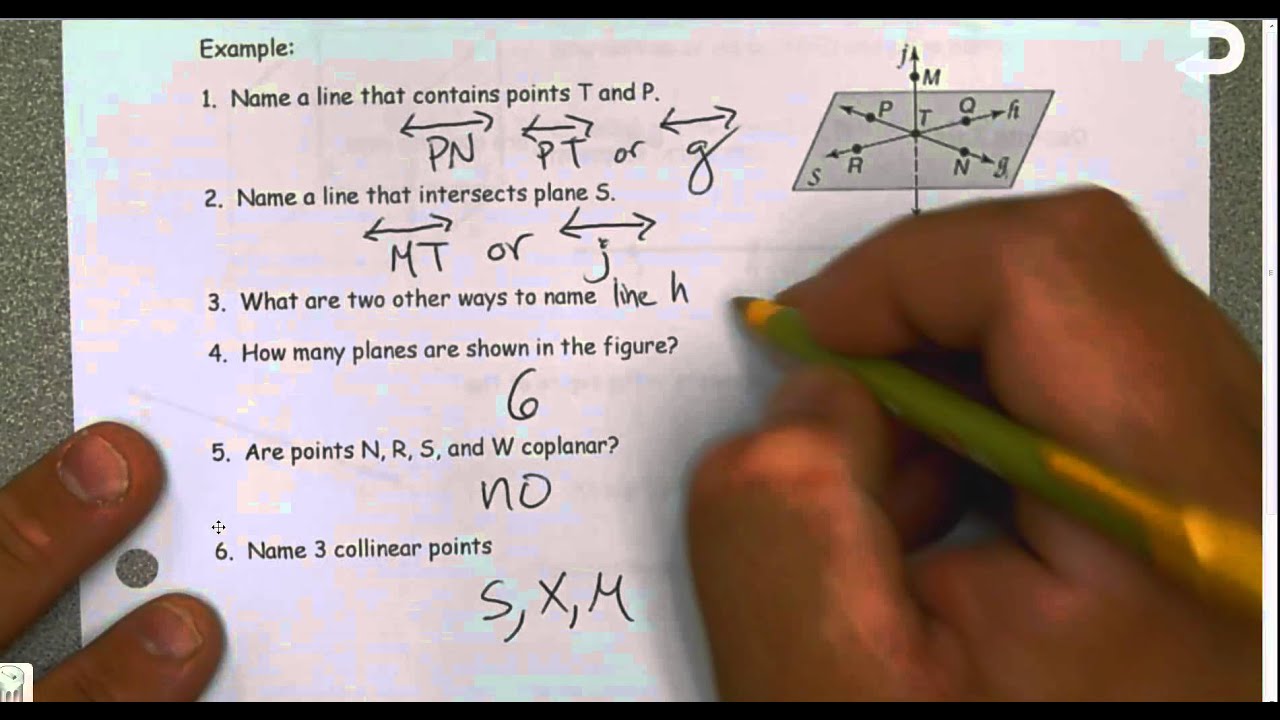
Image: learningnadeaudecerns.z21.web.core.windows.net

FAQ

Here are some frequently asked questions about Lesson 11.5 Practice A:

• Q: Is it necessary to memorize all the postulates and theorems for congruent triangles? A: It’s highly recommended. Being familiar with them will make solving problems much faster. But remember, understanding the concepts behind them is even more important. Practice applying them and you’ll naturally memorize them along the way.

<li><strong>Q:  Why is it important to learn about congruent triangles? </strong>  A: Congruent triangles are fundamental building blocks in geometry.  They are used in various applications, from solving geometric problems to understanding real-world situations, such as architectural designs and engineering structures</li>

Lesson 11.5 Practice A Geometry Answers

Conclusion

Lesson 11.5 Practice A geometry answers provide a platform for deepening your understanding of congruent triangles, crucial concepts in geometry. By mastering these concepts and practicing the problems, you’ll develop the skills to confidently tackle similar problems in the future. Are you ready to embrace the challenge and unlock the secrets of congruence?

Let me know if you have any specific questions or challenges related to Lesson 11.5 Practice A. I’m here to help you achieve your geometry goals!