2-4 Study Guide and Intervention – Writing Linear Equations

Remember that frustrating moment in algebra class when you felt utterly lost trying to decipher those cryptic equations? I certainly do. The concept of linear equations seemed like an impenetrable wall, but it didn’t have to be. Thanks to the insightful “2-4 Study Guide and Intervention” and a little bit of practice, I finally conquered my fear of linear equations. This resource became my secret weapon, helping me tackle the intricacies of equations and master the art of solving for the unknown.

Image: www.tessshebaylo.com

You’re about to embark on a journey to unravel the mysteries of linear equations, and just like I discovered, it’s a path that’s far less intimidating than it might initially seem. By delving into the “2-4 Study Guide and Intervention” we’ll uncover the core elements of linear equations, explore how to write them, and learn to confidently solve them. Ready to embrace the power of linear equations? Let’s get started!

Understanding Linear Equations: The Building Blocks of Relationships

Linear equations are like the blueprints of relationships between variables. They describe how one variable changes in relation to another, often forming a straight line when plotted. This makes them incredibly useful for analyzing and predicting trends in real-world scenarios. Imagine you’re tracking the growth of a plant. You notice it grows a certain amount each day. Linear equations can capture this relationship, allowing us to predict the plant’s height at any given time.

The beauty of linear equations lies in their simplicity and versatility. They can represent countless real-world phenomena, from calculating the cost of a phone plan based on minutes used to determining the distance traveled on a road trip. Understanding linear equations unlocks a whole new level of comprehension and predictive power.

Diving into the Structure of Linear Equations

The Essential Elements

At their core, linear equations are composed of a few key elements:

• Variables: These are the unknown quantities represented by letters, typically x and y. Think of them as placeholders waiting to be filled with specific values.
• Coefficients: Numbers multiplying the variables. Coefficients tell us how much each variable contributes to the overall equation.
• Constants: Numbers standing alone without any variables attached. Constants represent fixed values that remain unchanged.
• Equal Sign: The heart of the equation, indicating that the expression on the left side is equivalent to the expression on the right.

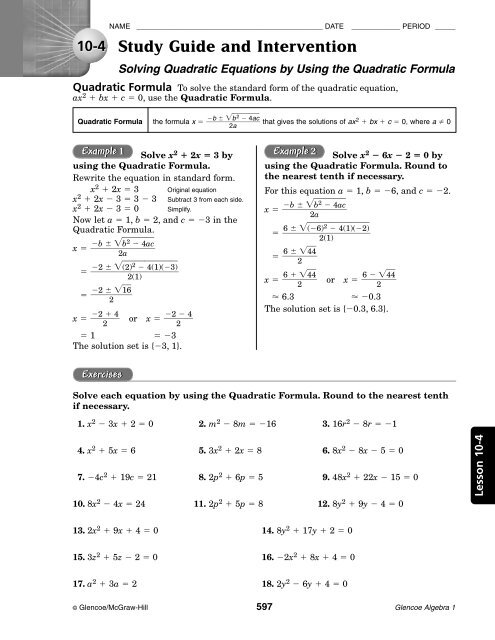
Image: www.pdfprof.com

Putting it Together: Examples of Linear Equations

Let’s illustrate these elements with some examples:

• y = 2x + 3: Here, ‘y’ and ‘x’ are the variables. The coefficient of ‘x’ is 2, indicating that ‘y’ increases twice as fast as ‘x’. The constant ‘3’ represents a starting value.
• 2x – 3y = 6: Here, we have two variables, ‘x’ and ‘y’, with their respective coefficients. The constant ‘6’ is a fixed value that influences the equation’s solution.

The Art of Writing Linear Equations

Writing linear equations involves transforming word problems into mathematical expressions. It’s like translating a sentence into a different language. The “2-4 Study Guide and Intervention” provides a guide to help you bridge the gap between words and equations. It outlines a step-by-step process that breaks down complex problems into manageable chunks.

Key Strategies for Writing Equations

• Identify the Variables: Determine which quantities are unknown and represent them with letters. For example, let ‘x’ represent the number of hours worked and ‘y’ represent the total earnings.
• Define the Relationship: Analyze the problem to understand how the variables are connected. Does one directly influence the other? Is there a constant value added or subtracted? For instance, if you earn $15 per hour, the equation would be y = 15x, where ‘y’ is the total earnings and ‘x’ is the number of hours worked.
• Include Constants: Consider fixed values or starting points that are involved in the problem. If you have a base salary of $500, the equation becomes y = 15x + 500, incorporating the constant ‘500’ as the initial salary.

Solving Linear Equations: Unlocking the Secrets

Solving a linear equation means finding the values of the variables that make the equation true. It’s like solving a puzzle, where you need to manipulate the equation to isolate the variable you’re interested in.

Methods for Solving Linear Equations

• Substitution: This involves isolating one variable in terms of the other and substituting that expression into the equation. Think of it as replacing one piece of the puzzle with its equivalent form.

  </li>

• Elimination: This method aims to eliminate one variable by combining equations in a way that cancels out its terms. Imagine removing a piece of the puzzle that isn’t needed to reveal the solution.

The “2-4 Study Guide and Intervention” guides you through these methods, providing clear examples and step-by-step solutions. It helps you decipher the logical steps involved in solving for unknowns. Don’t be afraid to practice! The more you work with these methods, the more comfortable and efficient you’ll become.

The “2-4 Study Guide and Intervention” in Action

“2-4 Study Guide and Intervention” isn’t just a static resource; it’s a tool that brings linear equations to life. It offers a variety of practice problems, both simple and complex, to test your understanding and build confidence. You can treat it like a personal tutor, providing guidance and feedback as you navigate through different scenarios.

Tips for Mastering Linear Equations

• Break it Down: Don’t be overwhelmed by complex problems. Break them down into smaller, more manageable steps. Focus on isolating one variable at a time.
• Practice Makes Perfect: The more you practice, the better you’ll become at identifying patterns and applying the appropriate methods. Don’t hesitate to revisit solved examples in the guide to solidify your understanding.
• Visualize the Relationship: Try plotting the equations on a graph. Understanding the visual representation of the relationship between variables can make it easier to solve equations.

FAQ

Q1: Why are linear equations so important?

A: Linear equations are essential because they allow us to model and analyze real-world relationships. They play a vital role in various fields, including physics, economics, finance, and engineering.

Q2: What if I’m stuck on a problem?

A: Don’t panic! Review the explanations and solved examples in the “2-4 Study Guide and Intervention.” Break the problem down into smaller parts. If you’re still stumped, seek help from your teacher or a tutor.

Q3: What are some common mistakes in solving linear equations?

A: Common mistakes include incorrect use of the order of operations (PEMDAS), forgetting to distribute multiplication across parentheses, and misplacing signs when combining terms.

2 4 Study Guide And Intervention Writing Linear Equations

Conclusion

Understanding linear equations opens up a world of possibilities in mathematics and beyond. The “2-4 Study Guide and Intervention” provides a valuable roadmap to help you master these equations, transforming them from intimidating obstacles into tools for analysis and problem-solving. Embrace the challenge, practice diligently, and watch as your confidence and understanding of linear equations soar.

Are you interested in learning more about the applications of linear equations in various fields? Share your thoughts and questions in the comments below!