Mastering Vectors – Demystifying Vector Problems with Solutions (Physics PDF)

Imagine you’re navigating a vast, uncharted sea. A gentle breeze nudges your ship, while the powerful ocean current tries to pull you in a different direction. To understand where you’re going, you need to consider both the wind’s force and the current’s pull. This intricate dance of forces, where direction matters as much as magnitude, is what the world of vectors is all about. Vectors, in essence, are arrows that point in specific directions, representing forces, velocities, or displacements – offering a powerful tool for understanding physics and the world around us.

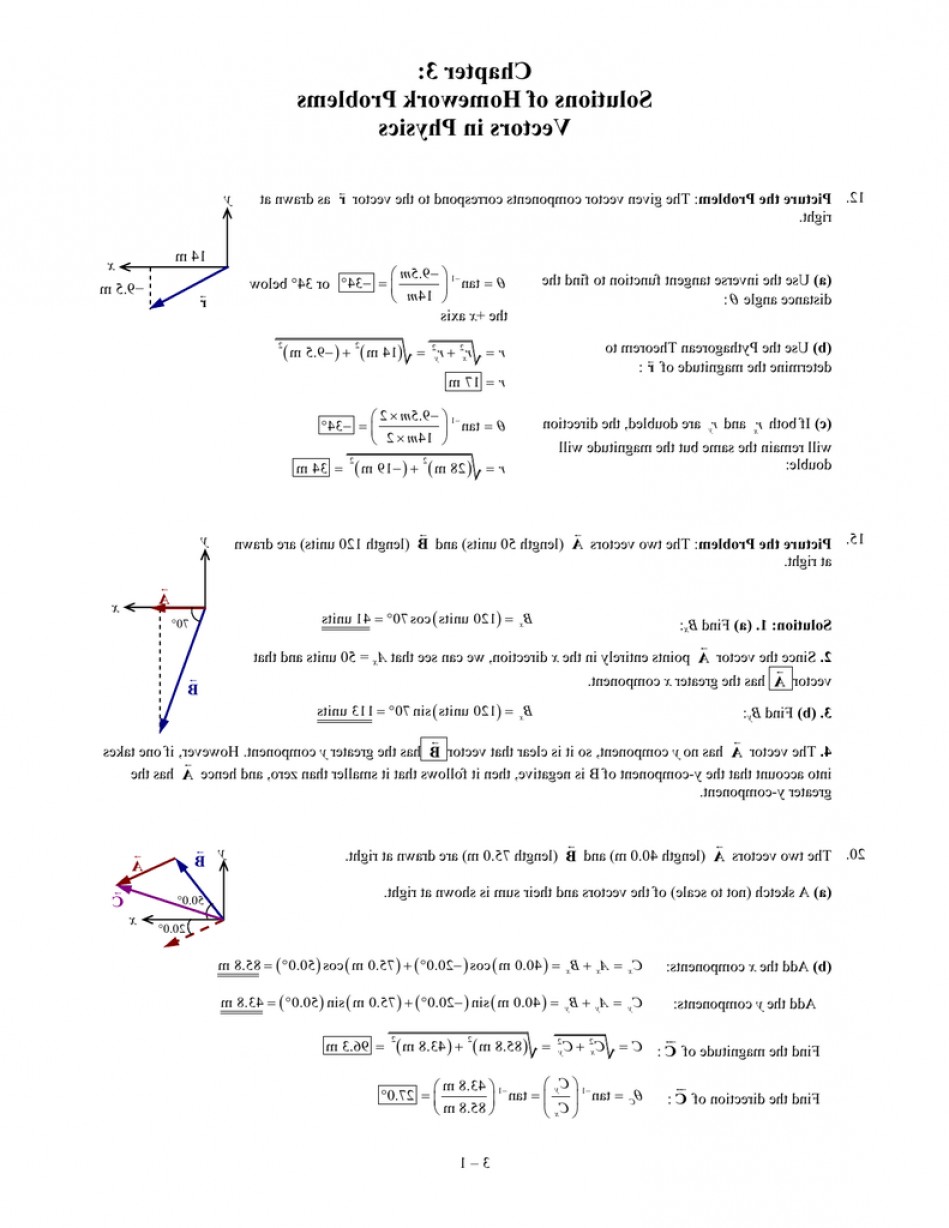
Image: vectorified.com

Today, we’ll embark on a journey to conquer the often intimidating realm of vector problems. This article will equip you with the knowledge and techniques to tackle vector problems with confidence, providing a clear understanding of how these essential tools work within physics. We’ll explore various types of vector problems, providing detailed solutions, and offering a downloadable PDF for you to practice at your own pace.

Diving into the World of Vectors:

At its core, a vector is a mathematical object that possesses both magnitude (length) and direction. Think of it as a force with a specific strength pushing in a particular way. When trying to understand the real-world interactions of these forces, we often face the challenge of combining and manipulating vectors. This is where vector problems come into play.

Types of Vector Problems and How to Approach Them:

Vector problems can be classified into several categories, each requiring specific techniques for solving them. Some common examples include:

• Adding Vectors: Imagine two forces pulling on a box, each in different directions. How would you calculate the overall force acting on the box? This is where vector addition comes into play.
• Subtracting Vectors: If you want to determine the difference in velocity between two objects, you would be dealing with vector subtraction.
• Multiplying Vectors by Scalars: Scalar multiplication involves changing the magnitude of a vector without altering its direction. Think of increasing or decreasing the strength of a force.
• Finding the Dot Product: The dot product provides information about the projection of one vector onto another. It’s used in applications like calculating work done by a force.
• Finding the Cross Product: The cross product yields a vector perpendicular to the original two vectors, used in concepts like calculating torque.

Essential Tools for Solving Vector Problems:

Several tools and techniques are used to make sense of these vector interactions:

• Graphical Methods: Visualizing vectors with arrows allows for intuitive understanding and can be helpful in solving simple problems. Imagine drawing two arrows representing forces, and then using the parallelogram method to find the resultant force.
• Trigonometric Methods: Using sine, cosine, and tangent functions can help break down vectors into their components, making it easier to manipulate them.
• Component Method: This method involves resolving vectors into their horizontal and vertical components, which can then be added or subtracted. It provides a systematic approach for complex problems.

Image: www.chegg.com

Example Vector Problems with Detailed Solutions:

Problem 1: Finding the Resultant Force

• Scenario: A person pushes a box with a force of 50 N east, while another person pushes the same box with a force of 30 N north. Find the net force acting on the box.
• Solution: We can use the Pythagorean theorem to find the magnitude of the resultant force:
  

  Resultant Force = √(50² + 30²) ≈ 58.3 N.
  To find the direction, we use trigonometry:
  tan θ = (opposite side) / (adjacent side) = 30 / 50
  θ = arctan (30 / 50) ≈ 31º.
  The net force is approximately 58.3 N at 31 degrees north of east.

Problem 2: Determining the Resultant Velocity

• Scenario: A boat travels at 15 m/s east relative to the water. The water is flowing at 5 m/s north. Find the boat’s velocity relative to the ground.
• Solution: The boat’s velocity relative to the ground is the vector sum of its velocity relative to the water and the water’s velocity. Again, we use the Pythagorean theorem:
  

  Resultant Velocity = √(15² + 5²) ≈ 15.8 m/s.
  The angle is found using:
  tan θ = 5 / 15
  θ = arctan (5 / 15) ≈ 18.4º.
  The boat’s velocity relative to the ground is approximately 15.8 m/s at 18.4 degrees north of east.

Mastering Vector Problems: Tips and Strategies:

• Visualize: Draw diagrams to represent the vectors and their components. This will help you understand the problem better and make it easier to solve.
• Break it Down: Resolve vectors into their components to simplify complex problems.
• Use the Right Tools: Choose the most appropriate method based on the problem’s nature (graphical, trigonometric, or component method).
• Practice, Practice, Practice: The key to mastering vector problems is consistent practice. Work through various examples and gradually increase the problem’s complexity.

Empowering Your Understanding: The Vector Problems with Solutions PDF:

To further enhance your learning, we have prepared a downloadable PDF containing a comprehensive collection of vector problems with detailed solutions. This resource will provide you with ample opportunities to practice and solidify your understanding of these critical concepts.

Vector Problems With Solutions Physics Pdf

Conclusion: Navigating the Vector Landscape:

Vectors, like the navigational forces of wind and current, play a vital role in understanding physics and other fields. By unraveling their intricacies, we gain a deeper appreciation for how forces interact and shape our world. We hope this article has equipped you with the tools and confidence to tackle vector problems with ease. Remember to practice, explore, and continue your journey of learning and discovery.