Remember those frustrating algebra problems we all encountered in school? The ones with variables and exponents that seemed like a cryptic foreign language? While those might have felt like a headache at the time, they were actually laying the foundation for understanding a fundamental concept in mathematics: polynomials. Polynomials are simply expressions consisting of variables and constants, combined using operations like addition, subtraction, and multiplication. This opens a world of possibilities—from describing the path of a ball thrown in the air to predicting the growth of a population. While they might appear intimidating at first, mastering the basic operations with polynomials is key to unlocking their power and unlocking other mathematical concepts.
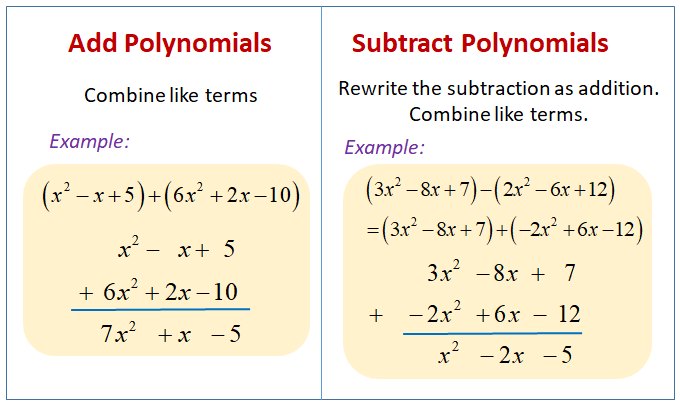
Image: worksheetzonececilia.z21.web.core.windows.net
Today, we’re diving into the core operations with polynomials: addition, subtraction, multiplication, and division. We’ll demonstrate these concepts with clear examples and step-by-step explanations to make them approachable. So buckle up and get ready to unlock the secrets of polynomials!
Understanding the Building Blocks: Monomials and Polynomials
Before we dive into operations, let’s first understand what makes up a polynomial. The smallest unit within a polynomial is a monomial, a single term that combines a coefficient (a numerical factor) and a variable raised to a non-negative integer exponent. For example, 3x2 is a monomial, where 3 is the coefficient, x is the variable, and 2 is the exponent.
A polynomial, as its name suggests, is simply a combination of these monomials, linked together by addition or subtraction. Think of polynomials as building blocks, where each monomial serves as a block, and the polynomial is the structure you construct with those blocks. For instance, 2x3 + 5x – 7 is a polynomial with three terms.
Essential Operations: Cracking the Code of Polynomials
1. Addition and Subtraction: Bringing Terms Together
Adding and subtracting polynomials is surprisingly straightforward. The key is to combine like terms—those with the same variable and exponent. Imagine you have two sets of blocks, and you need to combine them. You would only put blocks of the same type together, right? It’s the same with polynomials.
Let’s illustrate this with an example:
(2x2 + 3x – 1) + (x2 – 4x + 5)
To add these polynomials, we simply combine the corresponding like terms:
- Combine the x2 terms: 2x2 + x2 = 3x2
- Combine the x terms: 3x – 4x = -x
- Combine the constants: -1 + 5 = 4
Adding these individual results together, we get the final answer: 3x2 – x + 4

Image: lisaworksheets.com
2. Multiplication: Expanding the Possibilities
Multiplying polynomials is like constructing a more complex building. You need to carefully consider the interactions between each block. In the case of polynomials, we use the distributive property to multiply each term of one polynomial by each term of the other.
Take, for example, the multiplication of (x + 2) and (x – 3). We multiply each term in the first polynomial by each term in the second:
- x multiplied by x is x2
- x multiplied by -3 is -3x
- 2 multiplied by x is 2x
- 2 multiplied by -3 is -6
Combining these terms, we get: x2 – 3x + 2x – 6
After combining like terms, the final answer is x2 – x – 6.
3. Division: Separating into Smaller Parts
Polynomial division is slightly more intricate but still manageable. It uses a similar process to long division with numbers, but with variables and exponents added into the mix. This method helps us break down larger polynomials into simpler parts.
Let’s consider an example: (2x3 + 5x2 + 1) / (x + 2)
You start by setting up the division like traditional long division, focusing on the highest power term in each polynomial:
| x + 2 | / | 2x3 + 5x2 + 1 |
The next step is to divide the leading term of the divisor (x) into the leading term of the dividend (2x3). The result (2x2) goes above the line. We then multiply the divisor (x + 2) by the result (2x2):
| x + 2 | / | 2x3 + 5x2 + 1 | |
| 2x2 | 2x3 + 4x2 |
Subtracting these terms, we obtain:
| x + 2 | / | 2x3 + 5x2 + 1 | |
| 2x2 | 2x3 + 4x2 | ||
| x2 + 1 |
We then repeat the process with the new leading term (x2) and continue to divide and subtract until we reach a remainder. This process might involve multiple steps, but it systematically breaks down the polynomial division.
Mastering Polynomial Operations: Tips and Advice
Practice, Practice, Practice! As with most skills, the key to mastering polynomial operations is consistent practice. Work through numerous examples, starting with simple problems and gradually increasing complexity. Pay attention to the steps involved, and don’t be afraid to ask for help if you encounter any difficulties.
Utilize Online Resources: There are numerous online resources available to assist you in understanding and practicing polynomial operations. Websites, videos, and even interactive exercises can be valuable learning tools that make the process engaging and interactive.
FAQ: Common Questions About Polynomial Operations
Q: Why is it important to learn polynomial operations?
A: Polynomial operations are fundamental building blocks for higher-level math concepts, such as algebra, calculus, and even abstract algebra. They play a crucial role in various fields, including science, engineering, and economics.
Q: Are there any shortcuts for polynomial multiplication?
A: Yes, the FOIL method (First, Outer, Inner, Last) can be a helpful shortcut for multiplying two binomials. However, it’s crucial to understand the underlying principles of the distributive property, as it forms the basis of FOIL.
Q: How can I differentiate between monomials, binomials, and trinomials?
A: A monomial has only one term, a binomial has two terms, and a trinomial has three terms. These are all variations of polynomials, each with a specific number of terms.
5 1 Practice Operations With Polynomials
https://youtube.com/watch?v=H9x-2mAPj2w
Conclusion: Unlocking the Power of Polynomials
Understanding polynomial operations is a crucial step in mastering the language of mathematics. By combining practice, online resources, and a grasp of the fundamentals, you can unlock the power of these expressions and explore their vast applications in various fields.
Are you ready to delve deeper into the fascinating world of polynomials? Share your thoughts and questions in the comments below!



![Cyclomancy – The Secret of Psychic Power Control [PDF] Cyclomancy – The Secret of Psychic Power Control [PDF]](https://i3.wp.com/i.ebayimg.com/images/g/2OEAAOSwxehiulu5/s-l1600.jpg?w=740&resize=740,414&ssl=1)

